hasmiracle.blogg.se - Fourier of a bipolar square wave

#Fourier of a bipolar square wave series#

Savoy RL, McCann JJ (1975) Visibility of low spatial frequency sine-wave targets. Patel AS (1966) Spatial resolution by the human visual system. Olzak LA, Thomas JP (1986) Seeing spatial patterns, In: Boff KR, Kaufman L, Thomas JP (eds) Handbook of perception and human performance, vol I, chap 7. Mullen KT (1985) The contrast sensitivity of human colour vision to red-green and blue-yellow chromatic gratings. Hoekstra J, Goot DPA van der, Brink G van den, Bilsen FA (1974) The influence of the number of cycles upon the visual contrast threshold for spatial sine wave patterns. Vision Res 27:249–255įindlay JM (1969) A spatial integration effect in visual acuity. Greenlee MW, Magnussen S (1987) Higher-harmonic adaptation and the detection of square wave gratings.

Spatial luminance contrast sensitivity tests of macaque and human observers.

Valois RL de, Morgan H (1974) Psychophysical studies on monkey vision - III.

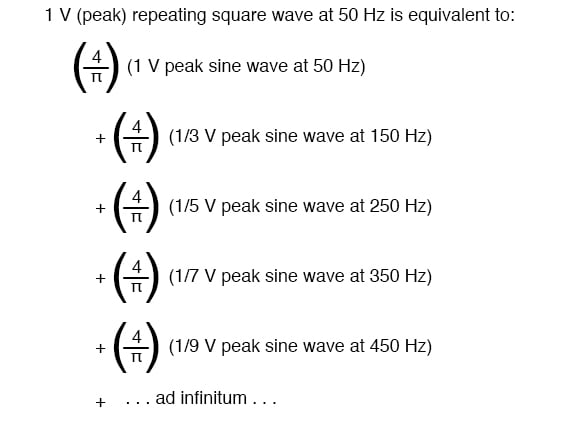
J Physiol (Lond) 204:283–298Ĭampbell FW, Robson JG (1968) Application of Fourier analysis to the visibility of gratings. Square wave gratings are better than sine wave greetings for studying resolution.Ĭampbell FW, Carpenter RHS, Levinson JZ (1969) Visibility of aperiodic patterns compared with that of sinusoidal gratings. Results with simulated BCs compare favorably with human and macaque psychophysics measuring contrast sensitivity. Amplitudes at all spatial frequencies are enhanced by increasing the number of cycles in the sine and square wave gratings. In general the harmonic with the maximum amplitude gives the best correlation with RI for the three stimuli. Resolution computed by the Fourier transform is compared with the resolution index (RI), which is a method for determining resolution based on two-point discrimination in the space domain. Due to the “honeycomb” packing of the cones and BC matrices Fourier transforms are computed row by row using a one-dimensional FFT. Simulated achromatic and chromatic sine and square waves, and a two-bar stimulus are used to activate the BCs. ) are needed to approximate the function this is because of the symmetry of the function.Fourier analysis is used to study resolution of images processed by the matrix of simulated red-center (BCR) and green-center (BCG) bipolar cells (BC) of the human central fovea.

As before, only odd harmonics (1, 3, 5.

There is no discontinuity, so no Gibb's overshoot.

Even with only the 1st few harmonics we have a very good approximation to the original function. Conceptually, this occurs because the triangle wave looks much more like the 1st harmonic, so the contributions of the higher harmonics are less.

#Fourier of a bipolar square wave series#

The amplitudes of the harmonics for this example drop off much more rapidly (in this case they go as 1/n 2 (which is faster than the 1/n decay seen in the pulse function Fourier Series (above)).

As you add sine waves of increasingly higher frequency, the approximation gets better and better, and these higher frequencies better approximate the details, (i.e., the change in slope) in the original function.

Note: this is similar, but not identical, to the triangle wave seen earlier. If x T(t) is a triangle wave with A=1, the values for a n are given in the table below (note: this example was used on the previous page). During one period (centered around the origin) The periodic pulse function can be represented in functional form as Π T(t/T p).